Non-ergodicity in Classical Harmonic Oscillator System

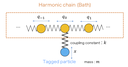Unlike the normal Langevin equation, the generalized Langevin equation, which deals with the memory effects, shows  various type diffusions depending on the memory function. Recently, the anomalous diffusion and the non-ergodicity have been actively studied in the terms of the generalized Langevin equation. There are, however, some confusions in the definition of the ergodicity and there are few analysis using physical models. We propose a new non-ergodic  model, which consists of harmonic oscillators, and analyze the model by the molecular dynamics, the exact diagonalization, and the analytical solution. We also reconsider the definition of the ergodicity, and clarify that the non-ergodicity observed in our model is caused by the localized mode.

• Fumihiro Ishikawa, Synge Todo, Localized Mode and Nonergodicity of a Harmonic Oscillator Chain, preprint: arXiv:1805.02923.